


Next: About this document ...
Lecture 15
Pressure and Fluidostatic
If I were to ask you which one is heavier, a kilogram of iron or a kilogram of feathers, you
would smile condescendengly and tell me that you are certainly not going to fall for such a
childish trick question. Still, we know that there is a big difference between a kg of iron and
one of feathers, and the difference is that you need a much larger quantity (i.e. a much larger
volume) of feathers to make up a kilo. We can quantify this difference by introducing the idea of
density: we call density 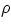 of a given substance the mass of a unit volume of it
Measuring
of a given substance the mass of a unit volume of it
Measuring 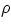 in kg/m3, is not very convenient, since it yields fairly large numbers for
ordinary materials (1 m3 is a relatively large volume), more commonly one expresses densities
in g/cm3 or in terms of specific gravity, i.e. the ratio between the density of the
substance and the density of water. Given that
in kg/m3, is not very convenient, since it yields fairly large numbers for
ordinary materials (1 m3 is a relatively large volume), more commonly one expresses densities
in g/cm3 or in terms of specific gravity, i.e. the ratio between the density of the
substance and the density of water. Given that 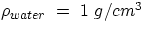 , specific gravity is
numerically equal to density measured in g/cm3.
, specific gravity is
numerically equal to density measured in g/cm3.
Question: isn't it strange that the density of water (at 40 to be precise) is exactly 1
g/cm3? Any idea why ?
Useful reminder for future doctors, pharmacists, etc :
1 liter = 1 dm3 = 1000 cm3 = 1000 cc
1 cc = 1 milliliter = 1 gram of water
It is important to remember that densities vary with pressure and temperature. The variation is not
very big for solids and liquids, but is quite sizeable for gases.
Pressure
We have already defined pressure as the force per unit surface. If I have a solid object
(e.g. a cylinder) of mass m resting on a table, and A is the area of contact, I can immediately see
that the pressure it exerts on the table is, if h is the height of the cylinder,
P = F/A = mg/A =
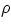
V g/A =
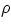
ghA/A =
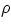
gh
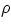 gh, with
gh, with 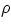 the liquid density and h its level above the
bottom.
the liquid density and h its level above the
bottom.
So far so good, but things become less intuitive if I put some object within the volume of the
liquid. Let's start by putting a flat fish like a flounder inthe water, and let's assume that it
is at rest; from what we just
said, its upper surface must feel a pressure 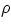 gh, where h is the depth of the flounder. But,
given that it is at rest, there must be an equal and opposite pressure acting on its lower surface.
gh, where h is the depth of the flounder. But,
given that it is at rest, there must be an equal and opposite pressure acting on its lower surface.
And the result would have been the same if I had taken a round fish like the balloon fish (picture
on page 401). If it is at rest, the same force per unit surface must be felt by every section of its
surface. We conclude that, at a given depth, the pressure exerted on a surface is independent of the
orientation of the surface. How can this come about? The answer is that, in a fluid, pressure is due
to the motion of the fluid molecules and their collisions against each other or against any
submersed object or even against the walls of the container. Think of the walls of a container full
of liquid : even though it is clear that they feel a pressure (if the walls weren't strong enough
the container would burst), this cannot be directly due to the weight of the liquid, since the
weight force is parallel to the side walls. The idea of fluid pressure being due to collisions with
fluid molecules is even more clear if we think of a gas. The walls of a gas filled balloon undoubtedly
are feeling a pressure, but this is obviously not due to the weight of the gas...
Still there are situations when the weight of a gas cannot be ignored : the column of air above us is
high enough, that its weight (and therefore its pressure) is not negligible. Can we estimate the actual
value of the pressure due to the atmosphere? We might think of using the expression P = 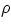 gh, with
h the height of the air column (some tens of kilometers), but this would not be straightforward, since
the density is not constant (you all know that there is "less air" at higher altitudes) since in a gas
density strongly depends on pressure.
gh, with
h the height of the air column (some tens of kilometers), but this would not be straightforward, since
the density is not constant (you all know that there is "less air" at higher altitudes) since in a gas
density strongly depends on pressure.
Atmospheric pressure is more easily measured, and this
was done for the first time by Evangelista Torricelli, a student of Galileo, who was asked to figure
out why one could pump water out of mines only up to a height of about 10 meters. The belief of the
time was that water pumps worked since they created a vacuum above the water surface, and water then
rushed in to fill the vacuum (Natura abhorret vacuum). Torricelli instead realized that the agent was
the atmospheric pressure, and in doing so he produced the first (mercury based) barometer.
Atmospheric pressure is quite useful, without it we could neither sip a drink through a straw, nor
use shaving cream....
For historical reasons , (atmospheric) pressure is measured im mm (or inches) of mercury as well as
in ...atmospheres. 1 atmosphere = 760 mm Hg . Can we convert atmospheres into pascals (N/m2)?
Another common unit for pressure is pounds per square inch (psi).
Absolute pressures can be measured with a Torricelli-like barometer, but often it is more convenient
just to measure the pressure excess (or defect) with respect to the atmospheric pressure: this
quantity is called gauge pressure. To measure gauge pressure one can use an open ended,
mercury filled, U-shaped tube, one end of which is connected to the pressure under measurement,the
other end to the open air (i.e. to atmospheric pressure). Differences in the height of the mercury
column in the two branches will indicate how much the pressure to be measured differs from atmospheric.
The standard sphygmomanometer measures artherial gauge pressure.
We have seen that the pressure in a fluid at a depth h, as due just to the weight of the fluid itself,
is given by
P =
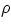
gh
 (where by h we mean the difference in
depth between positions P2 and P1) can be generalized to the case where there are additional
forces (i.e.
pressures) acting on the liquid. Suppose that we exert a force on a liquid (e.g. by means of a piston),
this force is going to be transmitted throughout the body of the liquid, and the expression above,
(where by h we mean the difference in
depth between positions P2 and P1) can be generalized to the case where there are additional
forces (i.e.
pressures) acting on the liquid. Suppose that we exert a force on a liquid (e.g. by means of a piston),
this force is going to be transmitted throughout the body of the liquid, and the expression above,
 , will still be valid, even if P1 now represents the whole pressure, due to
internal or external forces, acting at the point P1. And an immediate consequence is that, for two
locations at the same depth within the fluid volume, the pressure is the same, regardless of the shape
of the container. Formally, this effect is described by Pascal's principle:
, will still be valid, even if P1 now represents the whole pressure, due to
internal or external forces, acting at the point P1. And an immediate consequence is that, for two
locations at the same depth within the fluid volume, the pressure is the same, regardless of the shape
of the container. Formally, this effect is described by Pascal's principle:
the pressure applied at one point in one enclosed fluid is transmitted undiminished to every part
of the fluid and to the walls of the container
This property of fluids is exploited extensively in hydraulic lifts, etc.
Food for thought:
even though a hydraulic press allows to lift a heavy weight with little effort, there is no
violation of energy (and work) conservation. You can convince yourself of this by figuring out the
total displacement of the two sides of the hydraulic lift (and you can figure this out by
thinking in terms of the volume of fluid being displaced).
Archimedes' Principle
The story goes that, while floating in his bathtub, Archimedes saw the solution to a problem that his
"boss", the king of Syracuse, has posed to him, i.e. how to determine whether a crown was made of pure
gold without taking it apart. It is said that the thought occurred to him from noticing that the more he
immersed his body into the water, the more the water level rose. We can recognize two steps into Archimedes'
reasoning:
- 1.
- how to measure the volume (and therefore the density) of an object of irregular shape? Answer: fill
a container with liquid up to a certain level, fully immerse the body in it, and measure the new liquid level.
The height increase times the container's cross section wil give the volume of the object. Density of the
material can then be found from
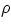 = m/V.
= m/V.
- 2.
- under what conditions will a body float or sink? Here the reasoning is the following : we start from
the previous result, i.e. an immersed body displaces an amount of liquid equal to its volume. When the body
is not in the liquid, the
liquid is in equilibrium, therefore each volume of water feels a force equal and opposite to its own weight.
When the body is immersed, nothing in principle changes, therefore the body will feel an upward force equal
to.... the weight of the amount of water it displaces.
Applying this result, we can immediately find the correct condition for floating or sinking :
a body immersed in a fluid (which could equally well be a liquid or a gas) is
subjected to its own weight, 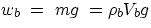 , and to the Archimedean push,
, and to the Archimedean push, 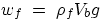 .The condition of equilibrium is then
.The condition of equilibrium is then
wb =
wf, i.e.
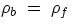
If the body's (average) density is less than that of the fluid the body will float, if it is more it will
sink. It is also straightforward to find what portion of a floating body is submersed : if we call Vs the
submersed portion for a body of total volume V, one has (can you prove it?):
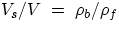
which is <1 when
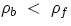
Fluidodynamics
In the most general case, the motion of a fluid is a rather complicated phenomenon (just think of white-water
rapids, or a boiling pot or the air and sewaves in a heavy storm, etc.). But in a large class of situations we
can make simplifying assumptions and obtain some very important results concerning the motion of fluids. In the
following we will assume that
- the fluid is incompressible, i.e. it has constant density (this is a very good assumption for
liquids, but it has to be handled carefully when dealing with gases)
- the fluid is non-viscous, i.e. there is no internal friction among neighbouring layers
- the fluid flow is such that the paths of different fluid particles do not intersect each other. This type
of flow is usually called laminar flow, and the non-laminar case is called turbulent.
The first result we can obtain when these conditions are (at least approximately) satisfied, is how the
velocity of a fluid changes as it goes through a path of variable width (more precisely, of variable
cross-section). To focus our thinking, let's consider a fluid flowing through a conduit of cross-section A.
If V is the volume of fluid that goes through a section of the conduit in the time 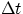 , one has
, one has
V = A x = A v
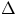
t
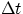 .But given that the total amount of fluid is conserved, and that the fluid is not compressible, this quantity has
to be the same everywhere along the fluid's path, i.e., if we take two positions of cross-section A1 and A2
we have
that shows how the speed of the flow increases as the fluid encounters a region of narrower cross section, or it
decreases in wider regions. This result goes under the name of continuity equation.
.But given that the total amount of fluid is conserved, and that the fluid is not compressible, this quantity has
to be the same everywhere along the fluid's path, i.e., if we take two positions of cross-section A1 and A2
we have
that shows how the speed of the flow increases as the fluid encounters a region of narrower cross section, or it
decreases in wider regions. This result goes under the name of continuity equation.
The next result, i.e. an expressions that relates pressure to velocity, is somewhat less intuitive, but it has
extremely far-reaching consequences and applications. To derive the result (known as Bernoulli's equation)
one has to apply to the fluid's motion the work-energy theorem, stating that the change in mechanical energy of
a system is given by the net work performed by non-conservative forces. If we consider a small volume of fluid
at positions 1 and 2, the change in mechanical energy is given by
(1/2mv12+mgy1) - (1/2mv22+mgy2)
The non conservative forces doing the work are effectively represented by the pressure in the fluid, and net
work will be done if there is a net pressure differential 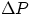 between the two faces of our fluid element.
If our small volume of fluid moves through a distance s, the net work done is
After a few simple step, one gets that the work-energy theorem implies
between the two faces of our fluid element.
If our small volume of fluid moves through a distance s, the net work done is
After a few simple step, one gets that the work-energy theorem implies
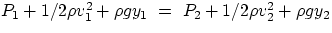
= constant
In the general case, each of the three terms in the expression above has to be taken into account. But it
is useful to consider a couple of special cases :
- 1.
- flow through a pipe of constant cross section. In this case the continuity equation ensures that
v1=v2, therefore we get
i.e. the same result we had already found for the static case
- 2.
- horizontal flow, y=const. In this case we get
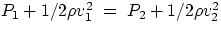
= constant
which tells us that in a fluid moving horizontally, an increase in velocity is accompanied by a decrease
in pressure.
Consequences and Applications:
- airplane lift
- curved balls
- indoor plumbing
- roof blowing off in strong winds (Problems 11.61, 11.67)
- Venturi meters (to measure fluid speed, e.g. speed of airplane w.r.t. surrounding air, or speed of fluid in
a pipe) (problem 11.69)
- prairie dog tunnel ventilation (Problem 11.59)
- etc.
Viscous Flow and Poiseuille Law : we will skip that (most of you are doing it this week in the Lab
anyway)



Next: About this document ...
Sergio Conetti
11/9/1999
![]() gh, with
h the height of the air column (some tens of kilometers), but this would not be straightforward, since
the density is not constant (you all know that there is "less air" at higher altitudes) since in a gas
density strongly depends on pressure.
gh, with
h the height of the air column (some tens of kilometers), but this would not be straightforward, since
the density is not constant (you all know that there is "less air" at higher altitudes) since in a gas
density strongly depends on pressure.