Lecture 9

Gravitational Potential Energy

Let us investigate what happens when the force doing work is the force of gravity. Specifically, let us examine a free-falling body, in the following scenarii :

1.

a body of mass m falls to the ground from a height h. Remembering that work is given by Force x displacement x cosine of the angle between the two, in this simplest case we have :

Force = mg, displacement = h, $\cos\theta =1 (\theta = 0^0)$

therefore the work is W = + mgh

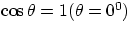
2.

starting from the same height h, the body is tossed up in the air, it reaches a certain height, and then eventually it falls down to the ground. We can divide the motion into three parts, 1) the trajectory from the starting point to the highest point h_max, 2) the trip back from h_max to the starting point and 3) the final trip from the starting point to the ground. One can immediately see that the work done by gravity in 1) is equal and opposite to that done in 2), so that the two contributions cancel out, and what is left is only the work to cover the original height h, i.e. W = mgh.

3.

the body is thrown horizontally from the same height h, and eventually it falls to the ground. We can analyze this situation in the following way : the total displacement is the combination of a horizontal and a vertical displacement. The horizontal displacement does not contribute to the work, since the force (gravity) is perpendicualr to it, so once again we get, for the total work, W = mgh

For simplicity we had assumed that the body was going from a height=h to a height = 0. In general, we could say that when a body goes from a height h_initial to a height h_final, the work done by gravity is

W = mg( h_initial - h_final)

Moreover, the work depends only on the initial and final positions, and not on the path followed to go from one to the other. A force obeying such a property is called a conservative force. For a while, gravity will be the only conservative force we will deal with (another important one we will study next year is the electrical force).

Let us examine our falling body further : as it falls from a height h, the body acquires a certain velocity v, i.e. a certain Kinetic Energy 1/2mv². Where does this energy come from? We have seen a while ago that the gain in kinetic energy of a body is given by the net work being done upon it. In this case the net work is done by gravity, and it is mgh. We can then write

1/2 mv² = mgh

which can be interpreted in the following way : at the beginning, the body, thanks to its "elevated position" has some potential energy (i.e., remembering the definition of energy, it has the "capability" of doing work). This potential energy mgh transforms itself into an equivalent amount of kinetic energy as the body falls to the ground.

Once again we have to be careful with the meaning of "h" : if we say that a body at a given height h has a gravitational potential energy mgh, we are entitled to ask : with respect to what should I measure h ? With respect to the ground ? But would the energy increase if I dig a hole under the object? Or if a body is sitting on a table on the second floor, should the energy be with respect to the floor of the (second floor) room or with respect to the ground floor ?

The answer is that, in any given situation, what comes into play is not an absolute value of potential energy, but the difference in energy between two levels h₁ and h₂. Therefore, it doesn't matter what the reference point is : for any given problem what will matter will be the difference in energy between h₁ and h₂, and the difference is always the same, regardless of what I choose as "0 level" (and, as usual, when doing differences pay attention to the signs).

Having said this, we can generalize the result obtained above : take a body that has an initial velocity v_i and an initial height (measured with respect to an arbitrary level) h_i. When moving under the sole influence of gravity to another level h_f its velocity changes to the value v_f. As usual the change in kinetic energy is given by the work of gravity, i.e. by the change in potential energy. In formulae :

1/2mv_f² - 1/2mv_i² = mgh_i - mgh_f, i.e.

1/2mv_f² + mgh_f = 1/2mv_i² + mgh_i

(E_kin + E_pot)_final = (E_kin + E_pot)_initial

But, in any given situation, we can choose the "initial" and "final" instant to be whichever ones we want. The above condition is then equivalent to

E_kin + E_pot = constant

This represents the Conservation of Mechanical Energy :

when a body moves solely under the influence of the gravitational force, the sum of its gravitational potential energy and its kinetic energy is constant

Notice that, in general, we should have said " ...under the sole influence of conservative forces...." and "...its potential energy....", but as I said before, for a while gravity is the only conservative force we deal with. Remember also that any force which is perpendicualr to the displacement (e.g. tension or normal force) does not need to be included in the energy balance equation since it does no work.

The principle of Mechanical Energy Conservation is enormously useful, and it facilitates the solution of a large class of problems (e.g. : what height will reach an object thrown vertically ? with what velocity will an object hit the ground and how will this depend on the actual trajectory? and what velocity will it have at any point in its trajectory? etc.)

So far so good, but what if we need to handle the (more realistic) situation where non-conservative forces (e.g. friction) are present ? We can almost guess the answer:

1.

Mechanical Energy is not constant anymore, but in fact

2.

the change in total Mechanical Energy is equal to the total work done by the non-conservative forces, i.e.

$W_{NC}~=~\Delta E_{kin}~+~\Delta E_{pot}$

and be sure to remember that each one of the three terms in the equation can be either positive of negative.

Power

In many situations, it is not enough to know how much work is being done, but also how fast is the work done. If I want to bring a 20 kg load up to the 5th floor (h=15 m), it does make a difference whether I do it in three minutes walking upstairs, or in 30 seconds with an elevator; but in either case the work is the same = mgh . We call Power the rate at which work is being done (or, which is the same, the work done in unit time):

Power = Work/time = W/t

No need to stress that, even if the rate of doing work is not constant, but W is the total work done, the above expression is still valid, but the quantity W/t represents the average power. The SI unit for power is joule/sec = watt. A common (historical) unit for power is.... the horsepower. 1 hp = 746 W = 0.746 kW . How much is your "personpower"

You can test it by running upstairs and measuring how long it takes you to gain a certain height : suppose you climb 10 meters (about 3 flights of stairs) in 10 seconds and your mass is 70 kg: the average power is ..... 80% of a horse. For sustained work, human beings are only worth 10-20% of a horse.

A unit you have often heard of is the kilowatthour (kWh) : do not be confused, kWh is a measure not of Power but of Energy (or Work). Here is the definition:

1 KWh is the work done (or the energy consumed) when doing work at the rate of 1 kW for 1 hour. Therefore :

1 kWh = 1 kW x 3600 sec = 1 kJ/s x 3600 s = 3600 kJ = 3.6 x 10⁶ J

Remembering also that work = force x displacement and that velocity = displacement/time, one can immediately find the alternative expression :

P = F v

which relates the (average) power involved when a force F acts on an object moving with an average speed v (notice that, since a net force is involved, speed will vary, so we can only approach the problem in terms of average speed; in any given problem, do not confuse average speed with final speed).

Work done by a Variable Force

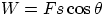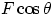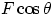We know that $W = F s\cos\theta$, and we know how to compute it for the case where $F\cos\theta$ does not change along s (or, alternatively, if it is the average value). For this simpler case, if we plot $F\cos\theta$ vs. s, we get a straight line. Given that W is given by the product of s x 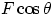$F\cos\theta$,we could say that W is given by the area coverd in the s vs. 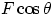$F\cos\theta$ plot.

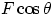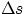This result can be generalized to the case of a non-constant Force (varying in magnitude and/or direction). In this case the s vs. $F\cos\theta$ plot will be an arbitrary curve. But if we divide it in small intervals $\Delta s$, we could say that, in each interval, the work is well approximated by the product 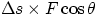$\Delta s\times F\cos\theta$ , where 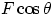$F\cos\theta$ is the value corresponding to each 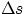$\Delta s$. 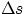And the smaller we make $\Delta s$ the better is our approximation. The total work will be the sum of all the small increments, and again will be given by the area covered by the s vs. 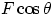$F\cos\theta$ curve.

(If you know calculus you will realize that what I am doing is an integral, if you don't, no need to worry, the idea should be clear enough as presented).