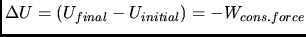Chapter 19

Electric Potential

To start, we should recall some very important facts concerning gravity and the relation between forces, work and energy:

• massive objects subjected to gravity possess a certain amount of gravitational potential energy, whose exact value depends on the position of the object with respect to the source of gravitational force (now we could even say the source of the gravitational field...). Near the surface of the earth, the potential energy of a mass m is $mgh$, where h is the height above an arbitrary reference level
• given that the choice of reference level is arbitrary, potential energy does not have an absolute value, we can only talk in terms of potential energy differences between two different positions
• when acting under the sole influence of gravity, the total mechanical energy of any object is constant (when a body is in free fall, we witness a continuous transformation of potential energy into kinetic energy, but their sum remains constant). A more general form of this statement would be: when under the sole influence of conservative forces the sum of potential + kinetic energy is a constant.
  
  reminder : a force is conservative when the work it does on a moving object depends only on the initial and final position, and not on the path followed. Gravity is an obvious example of a conservative force.
• work done by a force on a moving object is defined as force times displacement times the cosine of the angle between force and displacement, 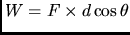$W = F\times d\cos\theta$. This implies that in general, when a force acts on a moving object, its work can be positive, negative or even zero (force perpendicular to displacement).
• whenever one or more forces do some work on a moving object, the result is some sort of energy transfer from one form to another. When you have both conservative and non-conservative forces :
  1. if the net force is zero, then the end result will be to change the object's potential energy. Example: lifting a mass m, with constant velocity, from 0 to a height h; the net work is zero, since the work done by the lifting force is equal and opposite to the work done by gravity. But, as there is a non-conservative force involved, i.e. the lifting force, then the (mechanical) energy of the object changes from 0 to $mgh$. Notice that this does not violate energy conservation, since the lifting force must get its energy from somewhere, either your muscles, or some engine.
  2. if on the other side, some net work is done on the body, the body will also increase its kinetic energy. Example: a plane climbing with increasing velocity; in this case, the work done by the engine both overcomes the force of gravity and gives some extra (kinetic) energy to the plane.

     In either case, there is a relation between the work done by the conservative force and the change in potential energy. Specifically, if U is the potential energy, one has

     $\Delta U = (U_{final}-U_{initial})=-W_{cons. force}$

We have seen that there are similarities between electricity and gravity. It is then logical to believe that an electric charge, when positioned within an electric field (i.e. when under the influence of some configuration of net charges) will possess a certain amount of electric potential energy. How can we be sure of that? Well, if we have a charge in an electric field and let it free, the charge will move under the influence of the force-field. But (motion x force) means work; by being in the field, the charge has the potential of doing work, i.e it has potential energy...

It would be nice to obtain a simple relation for the electric potential energy (something similar to $mgh$) but, when dealing with electricity, things are not so simple, for the following reason:

we should not forget that $mgh$ is an approximate expression, that assumes that, as a mass moves through the earth gravitational field, the strength of gravity remains constant. As we rememeber, the strength of gravity, on earth, depends on (the inverse square of) the distance from the center of the earth. As long as our changes in altitude are small with respect to the earth radius, then we can assume that gravity is constant and apply the approximate expression $mgh$. But, in general, such an approximation will not be possible when dealing with electricity, since, in most cases, changes in position of the charges under exam will not be negligible when compared to the distance between charges. Consequently, we will not be allowed to use an expression that assumes constant force. Still, we can examine some simple situations:

1. parallel plate capacitor: we have seen that between two parallel conducting plates charged with equal and opposite charges there is a constant electric field (given by $E=\sigma /\epsilon_{0}$). But having a constant field means that a charge will feel a constant force anywhere between the plates, and this is then a situation we can handle. What will be the difference in electric potential energy for a (negative) charge q when near the positive plate vs. being near the negative plate? To answer the question we must estimate the work needed to move the charge from the positive to the negative plate (the gravitational equivalent would be moving uphill). If d is the plate separation, we have
   $W=F\times d = E q d = (\sigma /\epsilon_{0}) q d$
   which then gives the value of the difference in electric potential energy the charge q has at the negative vs. the positive plate of a capacitor. If I was to release my charge after having forced it to the negative plate, it would move from the negative to the positive plate under the effect of a constant force (therefore with constant acceleration). In doing so, all of its potential energy would eventually be transformed into kinetic energy.
   
   Example :
   a)Let A, q and d be the area, charge and width of a parallel plate capacitor. If an electron is released with zero initial velocity near the negative plate, what will its velocity be when it reaches the positive plate?
   
   
   
   b)Answer question a) in terms of voltage difference between the plates
   
   
   
   c) Answer question a) using eV's
2. electric field due to a single positive charge Q. What will be the electric potential energy of another charge +q, positioned at a distance r from Q? To find the answer, we first need to choose our zero-energy reference level. The standard choice is to set the potential energy to zero when the charge separation is infinitely large. To estimate the potential energy of q at a distance r from Q, I then need to calculate the work required to move q from infinity to r. But this case is not as simple as the previous one, since, as q moves towards Q, the electric force is not constant but it keeps growing. The problem can be solved using calculus, with the answer :
   $W = kqQ/r$
   From this, we can also infer that the difference in electric potential energy of a charge q between two different positions, at respective distances $r_1$ and $r_2$ from Q is
   $\Delta E^{pot} = E^{pot}_{1}-E^{pot}_{2} = kqQ(1/r_{1}-1/r_{2})$

Electric Potential

In spite of the fact that Electric Potential Energy is an important concept, we are not going to encounter it too often in the future. Instead, we will mostly deal with a related quantity, the Electric Potential, also referred to as Electric Voltage.

DO NOT CONFUSE ELECTRIC POTENTIAL ENERGY WITH ELECTRIC POTENTIAL

they are related to each other but they are not the same thing. Here is how it goes:

we have already seen that, when dealing with electricity, it is more useful to think in terms of electric fields rather than electric forces, and we have defined the field vector as the force acting on a unit charge (or, which is the same the force acting on a charge divided by the charge's magnitude).

Similarly, rather than dealing with electric potential energy, we introduce a new quantity, the electric potential, defined as the (electric) potential energy of a given charge, divided by the charge magnitude, or, equivalently, the potential energy of a unit charge.

V = EPE/q

As for potential energies, electric potentials are meaningful only in relative terms, i.e. as differences between two different locations in an electric field. Based of the definition just given, we can say that the potential difference between two points is the difference in potential energy that a unit charge would have in the two locations or, which is the same, it is the work required to move a unit charge from one point to the other. Electric potentials are measured in volts and from our definition it follows that

1 volt = 1 joule/1 coulomb

From the results we had obtained earlier we can immediately obtain the expression for the potential in some simple cases:

1. the potential difference between the plates of a plane condenser with charge density $\sigma$ and plate separation $d$ is
   $\Delta V = (\sigma qd/\epsilon_{0})/q = \sigma d/\epsilon_{0}$
   But let's not forget that $\Delta V$ = work to move a unit charge from one plate to the other, therefore
   $\Delta V = E\times (q=1)\times d = E\times d$
   where E is as usual the magnitude of the electric field inside the capacitor. This result shows that it would be legitimate to measure field strengths in volts/meter (and in fact this is the more customary way to express a field strength). But earlier we were measuring fields in newton/coulomb. Are the two set of units equivalent?
2. the potential at a distance r from a point charge Q is
   $V = kQ/r$

In this last expression one must take into account the sign of the charge: a positive Q charge will generate a positive potential in the region surrounding it, while a negative charge will give origin to a negative potential. When dealing with a single charge we also have that the difference in potential between two positions $r_1$ and $r_2$ is

$\Delta V = kQ (1/r_{1}-1/r{_2})$

If we have more than one charge, the total potential at any location is the (algebraic) sum of the potentials due to the individual charges. A more rigorous treatment would show that the most general expression relating field and voltage is $E = -\Delta V/\Delta s$, where the minus sign accounts for the facts that positive charges move from higher to lower potentials and the field direction shows the force acting on a positive charge. This expression can be used when dealing with variable fields, in which case it has to be evaluated over a suitably small $\Delta s$

Conceptual question 19.6: compare electric field and electric potential at the midpoint of two identical charges, for the cases of same sign or opposite sign.

More on units
If you move one electron in an electric field region from a 0 V location to a -1 V location, the electron will acquire a (potential) energy of $q_{e}\times V = 1.6\times 10^{-19} J$. Similarly, if you let one electron move, under the influence of a field, from a -1V to a 0 V position, the electron will undergo an energy transformation (from potential to kinetic ) of $1.6\times 10^{-19} J$. When dealing with atomic and sub-atomic phenomena, it is then convenient to introduce a new energy unit, i.e. the electron volt, defined as the energy involved when a charge equal to the electron charge moves across the potential difference of 1 volt. Be sure to understand that the electron-volt (and its multiples MeV, GeV, etc.) is just an energy unit like any other (i.e. joules, calories, Btu's, etc) and one has $1 eV = 1.6\times 10^{-19}$

Equipotential surfaces

Let us consider the parallel plate capacitor. We have seen that, between the plates, the electric field is constant and given by $E= V/d$, where d is the plate separation and V the voltage difference between plates. What is the voltage at an intermediate location d' ? To answer this, we need to estimate the work required to move a unit charge to the location d', i.e. $W = E\times d'\times (q=1)$. From this we see that, within the plate's spacing, all the points of a plane parallel to the capacitor planes have the same potential V=Ed', where d' is the plane's position. We call this plane an equipotential surface, and we notice that it has the following properties:

1. an equipotential surface is perpendicular to the field lines
2. no work is done by the electric field when a charge moves along an equipotential surface. Moreover, no net work is done when a charge follows an arbitrary path that begins and ends on the same equipotential surface.

(statements 1 and 2 are in fact related, one could show that 1 follows from 2 and viceversa) . Equipotential surfaces do exist for any possible field configuration, the rule to establish them would be to draw the lines that at every location are perpendicular to the field lines ( a computer could easily do that for any arbitrarily complicated field). Apart from the parallel plate capacitor, the next simplest case is the one of the field generated by a single charge: in this case equipotential surfaces are spheres centered on the isolated charge.

Another immediate conclusion is that the surface of a conductor, whether charged or uncharged, is equipotential. If this wasn't the case, free (positive) charges would migrate from regions of higher to lower potential energy, the same way that a free mass spontaneously falls to the region of lowest gravitational potential(negative charges would do the opposite, i.e. they would move towards higher potentials).

Capacitance

From what we have just learned we can conclude that, when we add some extra net charge to a conductor, upon reaching equilibrium the whole body of the conductor settles at some constant voltage. A legitimate question would be : what will this voltage be, for a given amount of net charge? This question is equivalent to asking: if we pour a certain amount of water in a container, what level will the water reach? The answer obviously depends upon the capacity of the container, i.e. something related to its size and shape. The same happens with electricity : when adding a certain amount of charge to a conductor, it settles at a voltage determined by the conductor's capacitance, i.e something related to its size and shape. We express this idea with the relation

V = Q/C

For a given charge, the larger the capacitance, the lower the voltage level. Conversely, if we bring a conductor to a fixed voltage, e.g. by connecting it to a battery, the larger the capacitance the more charge we can store on it.

Typical Problem : a charged and an uncharged conductor of given capacities are brought into contact and then separated. How will the total charge be distributed among the two conductors? And what will be their voltage?

(Key to the solution : when brought together, the two conductors become equipotential, and their voltage does not change after they are taken apart).

Capacitance is measured in farads, whose defintion is obviously:

1 farad = 1 coulomb/1 volt

Application: thinking of the earth as a large conductive sphere, what is its capacitance?

To answer the question we need an expression for the capacity of a sphere of given radius. We can obtain it by recalling that, when we have a charge q inside a hollow spherical conductor, an equal charge q is induced on the conductor's outer surface, and the field generated by this charge is identical to the one that would be generated by the point charge q inside the conductor. We can then also say that the voltage at the sphere's surface is the same as that produced by a point charge $V=kq/r$. Therefore

$C = q/V=r/k=4\pi\epsilon_{0}r$

Applying this to the earth would show that 1 farad corresponds to a rather large capacitance.

When dealing with capacitance, a very important case is the one we have already encountered a few times, i.e. the parallel plate capacitor, since this configuration allows to maximize the capacitance (i.e is capable of storing relatively large amounts of charge). We study the parallel plate configuration since it is the easiest one to handle, but be aware that, in general, a capacitor is any pair of conductors separated by an insulator and carrying equal and opposite amounts of charge. From what we have learnt, we can immediately obtain an expression for the capacitance of a parallel plate configuration, in terms of its geometry. We have seen that V = Ed, where d is the plate separation, and also $E=\sigma/\epsilon_0$, where $\sigma$ is the charge density, i.e. $\sigma$=q/A (A=area of the plates). We then have

$E = V/d = q/(A\epsilon_0)$, therefore $C = q/V = \epsilon_{0}A/d$

Dielectric constant

Even though we have not stated it explicitely, so far we have assumed that the space bewteen the plates was empty. The situation changes when an insulating material is inserted between the plates. As discussed in the textbook, the effect of the insulating material, referred to as dielectric, is to lower the value of the electric field in the capacitor gap (some of the field lines are "swallowed" by space charges forming at the edges of the dielectric). The reduction in field strength depends on the prpoerties of the material (air e.g. has hardly any effect, and is almost equivalent to vacuum) and is expressed in terms of the material's dielectric constant $\kappa$, defined as

$\kappa = E_{0}/E$

wher $E_0$ and E are respectively the field without and with dielectric (consequently $\kappa\geq 1$). When a dielectric is present, the expression for the parallel plate capacitance then becomes:

$C =\kappa \epsilon_{0}A/d$

showing that the inclusion of a dielectric has the effect of increasing the capacitance(therefore allowing to store more charge for a given voltage).

Finally, let us estimate how much energy is stored in a capacitor. If a capacitor is charged up to a voltage V by an amount of charge q, the work involved is given by $q\times V_{av}$, where $V_{av}$ is the average value of the voltage as the capacitor is being charged. But since the voltage varies linearly with charge, the average voltage is just the intermediate value between initial and final, i.e. $V_{av} = V/2$. We then have

Energy = $W = qV/2 = (CV)V/2 = CV^{2}/2$