chap23

Chapter 23

RCL circuits

When dealing with Alternating Currents, the presence of inductors and capacitors in the circuit generates all sorts of new behaviours. Let's first examine each situation separately :

AC circuit with resistance only : in this case the circuit behaves like a DC circuit. We have
$V(t) = V_{max}\sin(2\pi ft)$ , $I(t) = I_{max}\sin(2\pi ft)$
and also
$V_{rms} = I_{rms}R$
Two things to notice are
1. the rms current is independent of the AC frequency f
2. voltage and current are always in phase, i.e. when one has a maximum, a minimum or a zero, so does the other.
AC circuit with capacitance only : to start, let's notice that in a circuit with a capacitor there can be no DC current. If we connect a capacitor to a battery, there is some current while the battery is charging up the capacitor, but then the current stops. But what if we connect a capacitor to a source of Alternating Voltage? As the voltage reverses the capacitor sequentially charges, discharges and then charges up to the opposite polarity. Even though there is no current across the capacitor's gap, there is an alternating current in the circuit.

What about the relation between voltage and current across the capacitor's plates? If we remember the () expression for the charging of a capacitor, we notice that the charging up is faster at the beginning (i.e. when the voltage between plates is near zero) and it slows down as the voltage approaches its maximum value. In other words voltage and current are 90 $^{0} (= \pi /2)$ out of phase, with the current LEADING the voltage. This can be expressed as
$V(t) = V_{max}\sin(2\pi ft)$
$I(t) = I_{max}\sin(2\pi ft+\pi /2) = I_{max}\cos (2\pi ft)$
And what is the relation between and (or between and ) ? It could be seen that a capacitor behaves like a resistance with the effective resistance (which goes under the name of capacitive reactance given by
$X_{C} = 1/(2\pi f C)$ and $V_{rms} = I_{rms}X_{C}$
from this we see that
1. the "resistance" is inversely proportional to the capacitance
2. the "resistance" does depend (inversely) on the frequency of the voltage source ( a good way to remember this is to think in terms of DC current : the "frequency" of a DC current is zero, and in this case the capacitance presents infinite resistance to the current)
AC circuit with inductance only : we can immediately guess that the "resistance" to the current will be directly related to the frequency : a fast changing voltage induces an opposing emf, and the faster is the change the larger the effect. And in fact one could derive the result
$V_{rms} = I_{rms}X_{L}$ with $X_{L} = 2\pi f L$
being the inductive reactance. What about the relative phase of current through the inductor and voltage across it? Here, the reasoning is the following:

the self-induced emf depends on the rate of change of the current (remember: emf = -L $\Delta I/\Delta t$ ). The sine function changes slowly near a peak and quickly near a zero. Following this line of thought would show that the current is $\pi /2$ out of phase, with the current LAGGING the voltage. In formulae
$V(t) = V_{max}\sin(2\pi ft),$
$I(t) = I_{max}\sin(2\pi ft-\pi /2) = -I_{max}\cos (2\pi ft)$

Another important property of a purely capacitive or a purely inductive circuit is that in both cases the power consumption is zero. One could verify this by computing the overall value of P=IV over a whole cycle, and realizing that there are equal negative and positive contributions. In physical terms, during a positive power period the capacitor (or inductor) absorb electric power from the voltage source, during a negative cycle the energy is released back.

We are now ready to understand a (serial) circuit including R, C and L. To start, we can get an intuitive feeling for the dependence of current upon the frequency of the oscillating voltage:

when the frequency approaches zero, so will the rms current because of the effect of the capacitance C. Similarly, at very high frequencies, the rms current will be suppressed by the effect of the inductance L. If a (positive) function goes to zero at both ends of its range, there must be at least one intermediate value of the frequency for which the rms current reaches a maximum. As we will soon see, this is the condition of resonance.

To get a deeper insight in the behaviour of an RLC circuit we have to take into account the relative phases of currents and voltages across the various circuit elements. This is best done by introducing the concept of phasors.

To describe the behaviour of current or voltage in an AC circuit, we introduce a vector of magnitude equal to the maximum amplitude of current or voltage, and we let this vector rotate in the x-y plane with uniform angular velocity $\omega = 2\pi f$ . If I or V vary with the $\sin (2\pi f)$ behavior typical of AC, then, at all times, the instantaneous values of I and V are given by the projection of their phasor onto the y-axis. Remembering what we had learnt about the relative phases of I and V when dealing with Capacitances and Inductances, we can see that, in purely capacitive or inductive circuits, the V phasor will always make a 90 angle with the I phasor, with the V phasor preceding (following) the I phasor for inductive (capacitive) circuits.

What we want to obtain is the equivalent of Ohm's Law for an RLC circuit, i.e. an expression of the type

$V_{rms} = I_{rms}Z$

where the quantity Z, the "impedance", is the equivalent of the resistance for the case of Alternating Currents. From what we had learnt earlier, you might be tempted to conclude that $Z = R + X{_C} + X_{L}$ , but this is not correct since it does not take into account the fact that, at any point in the circuit, the voltages due to the R,L,C components are not in phase.A way to account for this, is to add vectorially the $V_{R},V_{C},V_{L}$ voltages: the magnitude of the vector sum will give us the maximum voltage in the circuit, and its projection on the y-axis will correspond to the voltage instantaneous value. It is fairly straightforward to get the vector's sum magnitude :

as $V_{L}$ and $V_{C}$ are both at right angle with respect to the current, but in opposite directions, they are always 180 from each other, and the magnitude of their sum is $\mid V_{L}-V_{C}\mid$ .
$V_{R}$ is always perpendicular to the $(V_{L}\leftrightarrow V_{C})$ line, so that the total magnitude is given directly by Pythagoras :
$V_{max}^{2} = V_{R}^{2} + (V_{L}-V_{C})^{2}$

But we also have $V_{R}=IR, V_{C}=IX_{C}, V_{L}=IX_{L}$ , therefore (after a couple of simple steps)

$V_{max} = I_{max}\sqrt{R^{2}+(X_{L}-X_{C})^{2}}$
and also
$V_{rms} = I_{rms}\sqrt{R^{2}+(X_{L}-X_{C})^{2}} = I_{rms}Z$

which is the expression we were looking for, showing that the total impedance of a serial RLC circuit is given by $Z = \sqrt{R^{2}+(X_{L}-X_{C})^{2}}$ .

A few more things we can learn from the phasor diagrams :

the relative phase angle $\phi$ between current and voltage is given by $\tan\phi = (X_{L}-X_{C})/R$
one could also see that 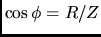$\cos\phi = R/Z$ , which is useful in determining the average power dissipation of an RCL circuit:

remembering that C and L do not consume any power, one has

$P = I_{rms}^{2}R = I^{2}_{rms}Z\cos\phi = I_{rms}I_{rms}Z\cos\phi = I_{rms}V_{rms}\cos\phi$

Problem : we knew how to estimate the voltage drop across each resistor when dealing with DC resistive circuits. What about an RLC AC circuit? The procedure is the following :

first compute the total impedance Z
next compute $I_{rms} = V_{rms}Z$
now you can do $V_{R} = I_{rms}R, V_{C}=I_{rms}X_{C}, V_{L}=I_{rms}X_{L}$

Example : $V_{rms}=90 V, f = 500Hz, R=25\Omega , L=30mH, C=12\mu F$

If we do the calculations, we find that the sum of the three voltage drops is not equal to 90 V. How can this be ?

The answer is that the rms voltages are defined to be always positive, while, at any instant, the real voltages are out of phase with each other and can be positive or negative. If we were to compute at any instant t the actual values of the voltages we would always get

$V_{R}(t)+V_{L}(t)+V_{C}(t) = V_{source}(t)$

Resonance

We can now verify the intuitive result we had discussed earlier, i.e. that in an RLC circuit there is a special value of the frequency for which the current is at a maximum (i.e. the impedance is at a minimum). The expression for the impedance shows that Z reaches its minimum value when $X_{C}=X_{L}$ , i.e. $f_{res} = 1/(2\pi\sqrt{LC})$

Notice that if there was no resistance (R=0), at the resonant frequency the current would become infinitely large. In reality resistances, even if small, can never be completely neglected, but it is still true that at the resonant frequency one can achieve very large values of current. And the smaller the resistance, the "sharper" the width of the resonance.

LC resonance is exploited in many electronics application. For ewxample, the tuning circuit of your radio is effectively an LC circuit: a variable capacitance is connected to the motion of the tuning dial, and by moving it you seek the LC resonant value corresponding to the broadcast frequency of the station you want to listen to.

Semiconductors and Transistors : read and retain as much as possible, even though the subject will not be included in the next tests

About this document ...

Next: About this document ...

Sergio Conetti 2003-03-11