3) A 100-pF (1 pF= F) capacitor is charged to 100 V. After the charging battery is disconnected, the capacitor is connected in parallel to another capacitor. If the final voltage is 30 V, what is the capacitance of the second capacitor? How much energy was lost?

Below we see the setup before and after the capacitors are connected.

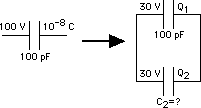
The initial charge on the capacitor is .

Therefore, the sum of the charges on the capacitors in parallel must equal this value as well.

[eq1]

We find the charge on the individual capacitors connected in series by

[eq2]

[eq3]

[eq2] and [eq3] plugged into [eq1] gives us

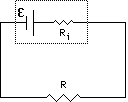
4) A battery of fixed emf and internal resistance is connected to a variable external resistance R. What must R be in order for the power delivered to the external resistor be maximal? (Remember that for an arbitrary function f(x), the maximum can be found by . Also for , one has )

Kirchhoff's loop rule gives us for the circuit shown below.

where

This fraction is zero if the numerator is zero, so we set

.

Dividing by gives us

BONUS PROBLEM: 25pts

5) A point charge ( ) is 10 cm from the middle of a thin metal needle 1 cm long (see figure). Estimate the charges on the ends of the needle required to maintain within the metal. (This is an approximation (which is not too bad considering that the length of the needle is much smaller than the distance to the charge) because is not uniformly zero in the needle).Hint: find a point in the needle where it is easiest to solve the problem.

The initial charge on the needle is zero, so the final charge (with Q involved) must also be zero. Any charge on the needle is going to reside at the points, so we have -q at the end closest to Q and +q at the end farthest from Q. The electric field at the center point of the needle is therefore

In order for , we must therefore have

(where we used )