Problem 2

 

2.) (15 points) A spherical insulator of radius a is completely surrounded by an uncharged conducting shell of inner radius b and outer radius c. We know that c > b > a >0. The insulator has a charge density $\rho$ spread uniformly throughout the material.
 
a)   What is the total charge on the insulator?
 
b)   Graph the electric field $\vec{E}$ as a function of the radius r, where $0 < r < \infty$. Label all interesting points to receive full credit.
 
c)   What is the value of the electric potential V in the region b < r < c?
 
Formulas you may or may not find useful... 

$\oint \vec{E} \cdot d\vec{A} = \frac{Q_{enclosed}}{\epsilon_{0}}$
$\vec{E} = -\nabla V$
$Volume_{sphere} = \frac{4}{3} \pi r^{3}$
$V_{b} - V_{a} = -\int_{r_{a}}^{r_{b}}\vec{E} \cdot d\vec{s}$

 

Answer:
 
a)
The total charge on the insulator, Q, is given by
$Q = \rho V$
where the volume of the insulator V, is given by
$V = \frac{4}{3} \pi a^{3}$
So, the total charge is given by
$Q = \frac{4}{3} \pi a^{3} \rho$.
The total charge is a constant, so the answer:
$Q = \frac{4}{3} \pi r^{3}$is incorrect because that implies that the total charge varies as a function of radius.

b)
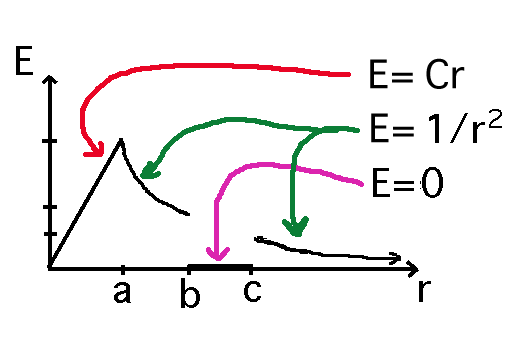
At r = 0, there is no inclosed charge, therefore, the electric field is zero. In the region, 0<r<a, the value for the enclosed charge varies as a function of radius.
$q_{inclosed} = \frac{4}{3} \pi r^{3} \rho$
$(E)(A) = \frac{q_{inclosed}}{\epsilon_{0}}$
$E (4\pi r^{2}) = \frac{4}{3} \pi r^{3} \rho \cdot \frac{1}{\epsilon_{0}}$
$E = \frac{\rho r}{3\epsilon_{0}}$
So, the electric field varies linearly with radius. In other words, its a straight line.
In the region, a<r<b, the total inclosed charge is a constant, so we have our usual $\frac{1}{r^{2}}$ field.
$E = \frac{a^{3} \rho}{3 \epsilon_{0} r^{2}}$
In the region, b<r<c, we are in an conductor, so the electric field is zero. In the region r>c, we return to our $\frac{1}{r^{2}}$ field. $E = \frac{a^{3} \rho}{3 \epsilon_{0} r^{2}}$
So, putting it all together, we have the following.
 
 

c)
To find the potential in the conductor, we have to find the potentia at the surface. The potential must be the same on both surfaces of the conductor and inside it. This value is not necessarily zero. We do know that
$V_{c}-V_{b} ~=~ \Delta V ~=~ -\int_{b}^{c} \vec{E} \cdot d\vec{r}$
$\Delta V = -\int_{b}^{c} 0 \cdot d\vec{r}$
$\Delta V = 0$
But, we don't need to find $\Delta V$, what we want is
$V_{c} - V_{b} = \Delta V = 0$,but what is V_c? To find that, we make use of the fact that the potential is zero at $\infty$.
$V_{c} - V_{\infty} = -\int_{\infty}^{c} \vec{E} \cdot d\vec{r}$
$V_{c} - 0 = -\int_{\infty}^{c} \frac{kQ_{total}}{r^{2}} dr$
$V_{c} = kQ_{total} (\frac{1}{c} - \frac{1}{\infty})$
$V_{c} = \frac{kQ_{total}}{c}$
So, the potential inside the conductor is given by
$V_{conductor} = \frac{a^{3}}{3 \epsilon_{0} c}$