University of Virginia
The cornerstone of the theory of special relativity is the Principle
of Relativity:
We shall see that many surprising consequences follow from this
innocuous looking statement.
Let us first, however, review Newton's mechanics in terms of frames
of reference.
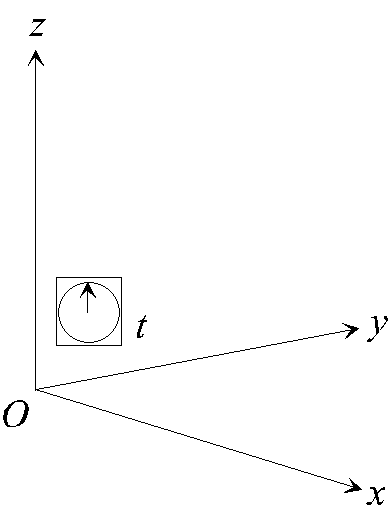
A "frame of reference" is just a set of coordinates
- something you use to measure the things that matter in Newtonian
mechanical problems - like positions and velocities, so we also
need a clock.
A point in space is specified by its three coordinates (x,y,z)
and an "event" like, say, a little explosion by a place
and time - (x,y,z,t).
An inertial frame is defined as one in which Newton's law
of inertia holds -- that is, any body which isn't being acted on
by an outside force stays at rest if it is initially at rest,
or continues to move at a constant velocity if that's what it
was doing to begin with. An example of a non-inertial
frame is a rotating frame, such as a carousel.
The "laws of physics" we shall consider first are those
of Newtonian mechanics, as expressed by Newton's laws of motion,
with gravitational forces and also contact forces from objects
pushing against each other. For example, knowing the universal
gravitational constant from experiment (and the masses involved),
it is possible from Newton's second law,
to predict future planetary motions with great accuracy.
Suppose we know from experiment that these laws of mechanics are
true in one frame of reference. How do they look in another frame,
moving with respect to the first frame? To find out, we have
to figure out how to get from position, velocity and acceleration
in one frame to the corresponding quantities in the second frame.
Obviously, the two frames must have a constant relative velocity,
otherwise the law of inertia won't hold in both of them. Let's
choose the coordinates so that this velocity is along the x-axis
of both of them.
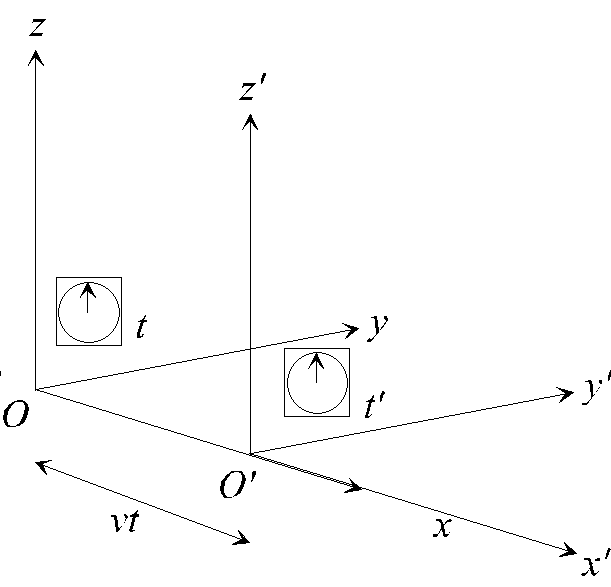
Notice we also throw in a clock with each frame.
Suppose S' is proceeding relative to S at speed v
along the x-axis. For convenience, let us label the
moment when O' passes O as the zero point of timekeeping.
Now what are the coordinates of the event (x,y,z,t)
in S'? It's easy to see t' = t -- we synchronized
the clocks when O' passed O. Also, evidently, y'
= y and z' = z, from the figure. We can also
see that x = x' +vt. Thus (x,y,z,t)
in S corresponds to (x',y',z', t' ) in S', where
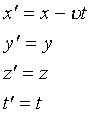
That's how positions transform - these are known as the Galilean
transformations.
What about velocities ? The velocity in S' in the x' direction
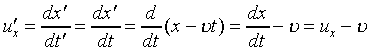
This is obvious anyway-it's just the addition of velocities formula
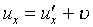
How does acceleration transform?
since v is constant.
That is to say,
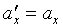
the acceleration is the same in both frames. This again is obvious -- the acceleration is the rate of change of velocity, and the velocities of the same particle measured in the two frames differ by a constant factor-the relative velocity of the two frames.
If we now look at the motion under gravitational forces, for example,
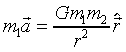
we get the same law on going to another inertial frame because
every term in the above equation stays the same .
Note that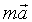 
is the rate of change of momentum -- this
is the same in both frames. So, in a collision, say, if total
momentum is conserved in one frame (the sum of individual rates
of change of momentum is zero) the same is true in all inertial
frames.
Physics 252 Home Page
Link to next lecture
Copyright © 1996 Michael Fowler