...pressure

In general, the specific heat is dQ/dT, or TdS/dT. This can be rewritten using the basic equation of thermodynamics (just energy balance), dE = TdS - pdV. At constant volume, dE = TdS and

$$C_{V}=T\left( \frac{\partial S}{\partial T}\right) _{V}=\left( \frac{\partial E}{\partial T}\right) _{V}$$

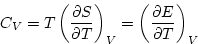
At constant pressure, it is convenient to work with the quantity H = E + pV, called the enthalpy. We find dH = dE + pdV + Vdp = TdS + Vdp, and so

$$C_{p}=T\left( \frac{\partial S}{\partial T}\right) _{p}=\left( \frac{\partial H}{\partial T}\right) _{p}$$

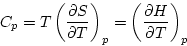
In an ideal gas, dE = C_VdT and pV = Nk_BT, so that dH = dE + d(pV) = (C_V + Nk_B)dT and C_p = C_V + Nk_B.