The Delta Function Potential

One limiting case of a square well is a very narrow deep well, which can be approximated by a delta function when the range of variation of the wave function is much greater than the range of the potential, so Schrödinger’s equation becomes

with l negative for an attractive potential.

The infinity of the d-function cannot be balanced by the finite right hand side, so the wave function must have a discontinuity in slope at the origin.

To find the ground state energy, note first that as a one-dimensional attractive potential there will be a bound state: any change in slope is sufficient to connect an exponentially increasing function coming in from -¥ to a decreasing one going to +¥, since the rates of increase and decrease can be arbitrarily slow.

Away from the origin, then, we can take the wave function to be

,

the energy of the state being .

The discontinuity in slope at the origin is just .

To match this with the d-function singularity, we integrate the Schrödinger equation term by term from -e to +e in the limit of e going to zero:

Note first that the right-hand side, having a finite integrand, must go to zero in the limit of e going to zero.

The d-function term must integrate to

The first term just gives the discontinuity in slope,

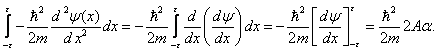
Schrödinger’s equation is therefore satisfied if (remembering l is negative for an attractive potential).

The energy of the bound state is

Exercise: rederive this result by taking the limit of a narrow deep well, tending to a d-function, with a cosine wave function inside.