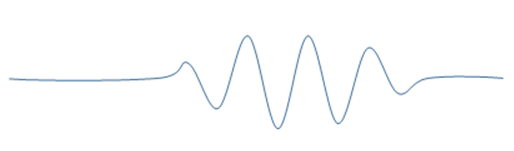
A "wavepacket" is an isolated sequence of wave oscillations, like the sound wave from a single brief note, or the ring moving outwards from a stone dropped in water, or the quantum wave representing a localized moving electron. It looks something like this:

The speed at which the packet moves is called the "group velocity". The individual crests within the packet move at the "phase velocity". For sound and light waves, these are the same, but for water waves and quantum electron waves they are different!
A simple way to illustrate this is to add two waves of slightly different wavelength—as we've done in this applet—so they periodically interfere, and in effect produce a string of wavepackets. We fixed the phase velocity at unity.
Vary the group velocity away from unity, and carefully follow one of the packets as it moves across the screen, then carefully follow one of the individual crests. You'll see the difference.
For quantum electron waves, the group velocity is twice the phase velocity, for water waves, it's half the phase velocity.
Watch the life and death of a single crest within a wavepacket! Which end does it disappear at?
Relevant Lecture for electron wavepacket.