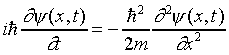.Michael Fowler
University of Virginia
Physics 252 Home Page
Link to Previous Lecture
The best way to gain understanding of Schrödinger's equation is to solve it for various potentials. The simplest is a one-dimensional "particle in a box" problem. The appropriate potential is V(x) = 0 for x between 0, L and V(x) = infinity otherwise -- that is to say, there are infinitely high walls at x = 0 and x = L, and the particle is trapped between them. This turns out to be quite a good approximation for electrons in a long molecule, and the three-dimensional version is a reasonable picture for electrons in metals.
Between x = 0 and x = L we have V = 0, so the wave equation is just
.
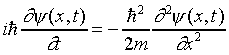A possible plane wave solution is
.
On inserting this into the zero-potential Schrödinger equation above we find E = p2/2m, as we expect.
It is very important to notice that the complex conjugate, proportional to 
, is not a solution to the Schrödinger equation! If we blindly put it into the equation we get
E = -p2/2m,
an unphysical result. However, a wave function proportional to 
gives E = p2/2m, so this plane wave is a solution to the equation.
Therefore, the two allowed plane-wave solutions to the zero-potential Schrödinger equation are proportional to 
and 
respectively.
Note that these two solutions have the same time dependence 
.
To decide on the appropriate solution for our problem of an electron in a box, of course we have to bring in the walls -- what they mean is that y = 0 for x < 0 and for x > L because remember |y |2 tells us the probability of finding the particle anywhere, and, since it's in the box, it's trapped between the walls, so there's zero probability of finding it outside.
The condition y = 0 at x = 0 and x = L reminds us of the vibrating string with two fixed ends -- the solution of the string wave equation is standing waves of sine form. In fact, taking the difference of the two permitted plane-wave forms above gives a solution of this type:
.
This wave function satisfies the Schrödinger equation between the walls, it vanishes at the x = 0 wall, it will also vanish at x = L provided that the momentum variable satisfies:
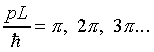
Thus the allowed values of p are hn/2L, where n = 1, 2, 3… , and from E = p2/2m the allowed energy levels of the particle are:
.
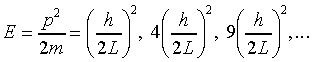Note that these energy levels become more and more widely spaced out at high energies, in contrast to the hydrogen atom potential. (As we shall see, the harmonic oscillator potential gives equally spaced energy levels, so by studying how the spacing of energy levels varies with energy, we can learn something about the shape of the potential.)
What about the overall multiplicative constant A in the wave function? This can be real or complex. To find its value, note that at a fixed time, say t = 0, the probability of the electron being between x and x + dx is |y |2dx or
.
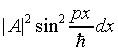The total probability of the particle being somewhere between 0, L must be unity -- so
.
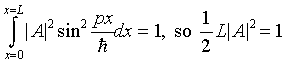Hence
.
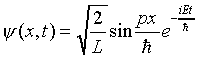When A is fixed in this way, by demanding that the total probability of finding the particle somewhere be unity, it is called the normalization constant.
Exercise: If an electron is confined to a one-dimensional box of length L, then the uncertainty in its position 
. Yet we claim in the eigenstates to know the momentum variable p exactly. How can you reconcile this with the uncertainty principle?
Notice that at a later time the probability distribution for the wave function
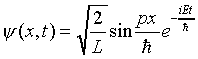
is the same, because time only appears as a phase factor in this time-dependent function, and so does not affect |y |2. A state with a time-independent probability distribution is called a stationary state. Such a state must have a definite energy -- as can be seen by constructing a solution to Schrödinger's equation by adding states of different energies, for example:
.
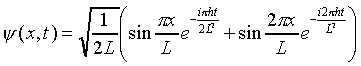(You can check the normalization constant at t = 0). For general x, the two terms in the bracket rotate in the complex plane at different rates, so their sum has a time-varying magnitude. That is to say, |y (x,t)|2 varies in time, so the particle must be moving around -- this is not a stationary state. However, it is not difficult to show that the total probability of finding the particle somewhere in the box remains unity.
The only way to prevent |y (x,t)|2 varying in time is to have all its parts changing phase in time at the same rate. This means they all correspond to the same energy. If we restrict our considerations to such stationary states, the wave function can be factorized
and putting this wave function into the Schrödinger equation we find
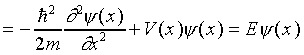
This is the time-independent Schrödinger equation, and its solutions are the spatial wave functions for stationary states, states of definite energy. These are often called eigenstates of the equation.
The values of energy corresponding to these eigenstates are called the eigenvalues.
Consider again the wavefunction for the lowest energy state of a particle confined between walls at x = 0 and x = L. The reader should sketch the wavefunction from some point to the left of x = 0 over to the right of x = L. To the left of x = 0, the wavefunction is exactly zero, then at x = 0 it takes off to the right (inside the box) as a sine curve. In other words, at the origin the slope of the wavefunction y is zero to the left, nonzero to the right. There is a discontinuity in the slope at the origin: this means the second derivative of y is infinite at the origin. On examining the time-independent Schrödinger equation above, we see the equation can only be satisfied at the origin because the potential becomes infinite there -- the wall is an infinite potential. (And, in fact, since y becomes zero on approaching the origin from inside the box, the limit must be treated carefully.)
It now becomes obvious that if the box does not have infinite walls, but merely high ones, y describing a confined particle cannot suddenly go to zero at the walls: the second derivative must remain finite. For non-infinite walls, y and its derivative must be continuous on entering the wall. This has the important physical consequence that y will be nonzero at least for some distance into the wall, even if classically the confined particle does not have enough energy to "climb the wall". (Which it doesn't, if it's confined.) Thus, in quantum mechanics, there is a non-vanishing probability of finding the particle in a region which is "classically forbidden" in the sense that it doesn't have enough energy to get there.
Physics 252 Home Page
Link to Next Lecture
Copyright ©1997 Michael Fowler