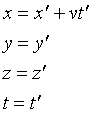
Michael Fowler
UVa Physics
Physics 252 Home Page
Link to previous lecture
We have already seen that Newtonian mechanics is invariant under the Galilean transformations relating two inertial frames moving with relative speed v in the x-direction,
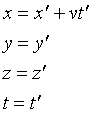
However, these transformations presuppose that time is
a well-defined universal concept, that is to say, it's the same
time everywhere, and all observers can agree on what time it is.
Once we accept the basic postulate of special relativity, however,
that the laws of physics, including Maxwell's equations, are the
same in all inertial frames of reference, and consequently the
speed of light has the same value in all inertial frames, then
as we have seen, observers in different frames do not agree
on whether clocks some distance apart are synchronized. Furthermore,
as we have discussed, measurements of moving objects are compressed
in the direction of motion by the Lorentz-Fitzgerald contraction
effect. Obviously, the above equations are too naïve! We
must think more carefully about time and distance measurement,
and construct new transformation equations consistent with special
relativity.
Our aim here, then, is to find a set of equations analogous to
those above giving the coordinates of an event (x,y,z,t)
in frame S, for example, a small bomb explosion , as functions
of the coordinates (x,y,z,t) of the same event measured
in the parallel frame S' which is moving at speed v
along the x-axis of frame S. Observers O
at the origin in frame S and O' at the origin in
frame S synchronize their clocks at t = t'
=0, at the instant they pass each other, that is, when the two
frames coincide. (Using our previous notation, O is Jack
and O' is Jill.)
To determine the time t' at which the bomb exploded in
her frame, O' could determine the distance of the point
(x',y',z') from the origin, and hence how
long it would take light from the explosion to reach her at the
origin. A more direct approach (which is helpful in considering
transformations between different frames) is to imagine O'
to have a multitude of helpers, with an array of clocks throughout
the frame, which have all been synchronized by midpoint flashes
as described in the previous lecture. Then the event -- the bomb
explosion -- will be close to a clock, and that local clock determines
the time t' of the event, so we do not need to worry about
timing a light signal.
In frame S', then, O' and her crew have clocks all along the x'-axis (as well as everywhere else) and all synchronized:
Now consider how this string of clocks appears as viewed by O
from frame S. First, since they are all moving at speed
v, they will be registering time more slowly by the usual
time dilation factor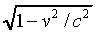 
than O's own
physically identical clocks. Second, they will not be synchronized.
From the clocks on a train argument in the last lecture, if the
clocks are L apart as measured by O', successive
clocks to the right (the direction of motion) will be behind by
Lv/c2 as observed by O.
It should be mentioned that this lack of synchronization as viewed from another frame only occurs for clocks separated in the direction of relative motion. Consider two clocks some distance apart on the z' axis of S'. If they are synchronized in S' by both being started by a flash of light from a bulb half way between them, it is clear that as viewed from S the light has to go the same distance to each of the clocks, so they will still be synchronized (although they will start later by the time dilation factor).
Let us now suppose that O' and her crew observe a small
bomb to explode in S' at (x',0,0,t'). In this section,
we shall find the space coordinates and time (x, y,
z, t) of this event as observed by O in the
frame S. (As above, S' moves relative to S
at speed v along the x-axis). In other words, we
shall derive the Lorentz transformations -- which are just the
equations giving the four coordinates of an event in one inertial
frame in terms of the coordinates of the same event in another
inertial frame. We take y',z' zero because they transform
trivially -- there is no Lorentz contraction perpendicular to
the motion, so y = y' and z = z'.
First, we consider at what time the bomb explodes as measured
by O. O''s crew found the bomb to explode at time
t' as measured by a local clock, that is, one located at
the site of the explosion, x'. Now, as observed by O
from frame S, O''s clock at x' is not
synchronized with O''s clock at the origin S'. When
the bomb explodes and the clock at x' reads t',
O will see O''s origin clock to read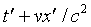 
.
What does O's own clock read at this point? Recall that
O, O' synchronized their origin clocks at the moment
they were together, at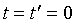 
. Subsequently,
O will have observed O''s clock to be running slowly
by the time-dilation factor. Therefore, when at the instant of
the explosion he sees O''s origin clock to be reading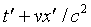 
,
he will find that the true time t in his frame is equal
to this appropriately scaled to allow for time dilation, that
is,
This is the first of the Lorentz transformations.
The second question is: where does O observe the
explosion to occur?
Since it occurs at time t after O' passed O,
O' is vt meters beyond O at the time of the
explosion. The explosion takes place x' meters beyond O',
as measured by O', but of course O will see that
distance x' as contracted to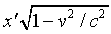 
since
it's in a moving frame.
Therefore O observes the explosion at point x given
by
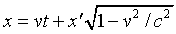
This can be written as an equation for x in terms of x',
t' by substituting for t using the first Lorentz transformation
above, to give
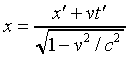
Therefore, we have found the Lorentz transformations expressing the coordinates (x,y,z,t) of an event in frame S in terms of the coordinates (x',y',z',t') of the same event in frame S':
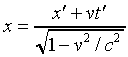
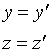
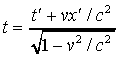.Notice that nothing in the above derivation depends on the x-velocity v of S' relative to S being positive. Therefore, the inverse transformation (from (x,y,z,t) to (x', y', z',t')) has exactly the same form as that given above with v replaced by -v.
Consider now the following scenario: suppose that as O' passes O (the instant both of them agree is at time t' = t = 0) O' flashes a bright light, which she observes to create an expanding spherical shell of light, centered on herself (imagine it's a slightly foggy day, so she can see how the ripple of light travels outwards). At time t', then O' (or, to be precise, her local observers out there in the frame) will see a shell of light of radius ct', that is to say, they will see the light to have reached all points (x', y', z') on the surface
Question: how do O and his observers stationed throughout
the frame S see this light as rippling outwards?
To answer this question, notice that the above equation for where the light is in frame S' at a particular time t' can be written
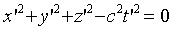,and can be thought of as a surface in the four dimensional (x', y', z', t') space, the totality of all the "events" of the light reaching any particular point. Now, to find the corresponding surface of events in the four dimensional (x, y, z, t) space, all we have to do is to change from one set of variables to the other using the Lorentz transformations:
.
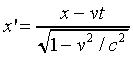
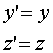
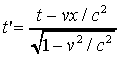On putting these values of x', y', z', t'
into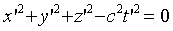 
, we find that the corresponding
surface of events in (x, y, z, t)
space is:
.
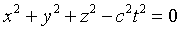This means that at time t, O and his observers in
frame S will say the light has reached a spherical surface
centered on O.
How can O' and O, as they move further apart, possibly
both be right in maintaining that at any given instant the outward
moving light pulse has a spherical shape, each saying it is centered
on herself or himself?
Imagine the light shell as O' sees it -- at the instant t' she sees a sphere of radius r', in particular she sees the light to have reached the spots +r' and -r' on the x' axis. But from O's point of view the expanding light sphere does not reach the point +r' at the same time it reaches -r' ! (This is just the old story of synchronizing the two clocks at the front and back of the train one more time.) That is why O does not see O''s sphere: the arrival of the light at the sphere of radius r' around O' at time t' corresponds in S to a continuum of different events happening at different times.
We found above that for an event (x', y', z',
t') for which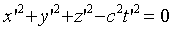 
, the coordinates
of the event (x, y, z, t) as measured
in the other frame S satisfy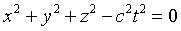 
.
The quantity x2 + y2 + z2
- c2t2 is said to be a Lorentz
invariant, it doesn't vary on going from one frame to another.
A simple two-dimensional analogy to this invariant is given by considering two sets of axes, Oxy and Ox'y' having the same origin O, but the axis Ox' is at an angle to Ox, so one set of axes is the same as the other set but rotated. The point P with coordinates (x,y) has coordinates (x', y') measured on the Ox'y' axes. The square of the distance of the point P from the common origin O is x2 + y2 and is also x'2 + y'2, so for the transformation from coordinates (x,y) to (x', y'), x2 + y2 is an invariant. Similarly, if a point P1 has coordinates (x1,y1) and (x1', y1') and P2 has coordinates (x2,y2) and (x2',y2') then clearly the two points are the same distance apart as measured with respect to the two sets of axes, so
.
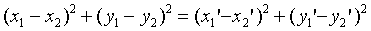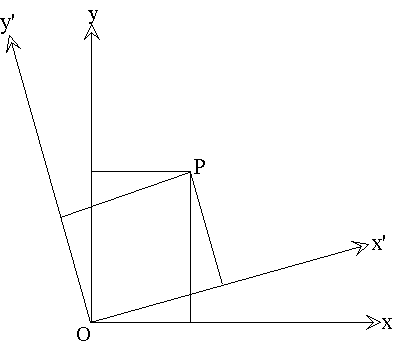
This is really obvious-the distance between two points in an ordinary
plane can't depend on the angle at which we choose to set our
coordinate axes.
The Lorentz analog of this, dropping the y,z coordinates,
can be written
,
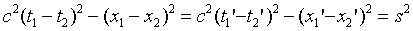say, where s is some sort of measure of the "distance" between
the two events (x1,t1) and (x2,
t2).
This s is sometimes called the "space-time interval". The
big difference from the two-dimensional rotation case is that
s2 can be positive or negative. For s2
negative, the "distance" itself would be imaginary, so obviously this is rather a
formal concept! In practice, as we shall see below, it's
clear enough what to do in any particular case -- the cases of spacelike and timelike
separated events are best dealt with separately, at least to begin with.
Consider first two events simultaneous in frame S', so
t1' = t2'. They will not be
simultaneous in frame S, but they will satisfy
.
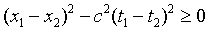We say the two events are spacelike separated. This means
that they are sufficiently removed spatially that a light signal
could not have time between them to get from one to the other,
so one of these events could not be the cause of the other.
The sequence of two events can be different in different frames
if the events are spacelike separated. Consider again the starting
of the two clocks at the front and back of a train as seen from
the ground: the back clock starts first. Now imagine viewing this
from a faster train overtaking the clock train -- now the front
clock will be the first to start. The important point is that
although these events appear to occur in a different order in
a different frame, neither of them could be the cause of the other,
so cause and effect are not switched around.
Consider now two events which occur at the same place in frame S' at different times, (x'1,t'1) and (x'2,t'1). Then in frame S:
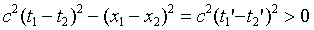.These events are said to be timelike separated. There is no frame in which they are simultaneous. "Cause and effect" events are timelike separated.
Let us try to visualize the surface in four-dimensional space described by the outgoing shell of light from a single flash,
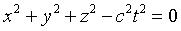.It is helpful to think about a simpler situation, the circular ripple spreading on the surface of calm water from a pebble falling in. Taking c here to be the speed of the water waves, it is easy to see that at time t after the splash the ripple is at
.
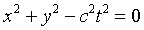Now think about this as a surface in the three-dimensional space
(x, y, t). The plane corresponding to time
t cuts this surface in a circle of radius ct. This
means the surface is a cone with its point at the origin. The
four-dimensional space flash-of-light surface is not so easy to
visualize, but is clearly the higher-dimensional analog: the plane
surface corresponding to time t cuts it in a sphere instead
of a circle. This surface is called the lightcone.
We have stated above that the separation of a point P (x,
y, z, t) from the origin is spacelike if
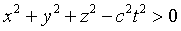and timelike if 
.
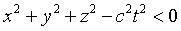It is said to be lightlike if 
.
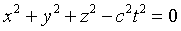Points on the light cone described above are lightlike separated
from the origin. To be precise, the points corresponding to an
outgoing shell of light from a flash at the origin at t
= 0 form the forward light cone. Since the equation depends
only on t2, there is a solution with t
negative, the "backward light cone", just the reflection
of the forward light cone in the plane t = 0.
Possible causal connections are as follows: an event at the origin (0, 0, 0, 0) could cause an event in the forward light cone: so that is the "future", as seen from the origin. Events in the backward light cone -- the "past" -- could cause an event at the origin. There can be no causal link between an event at the origin and an event outside the light cones, since the separation is spacelike: outside the light cones is "elsewhere" as viewed from the origin.
Physics 252 Home Page
Link to next lecture
Copyright ©1996 Michael Fowler