Fluids will invariably swirl around, and it is often useful to
characterize the amount of swirling. This is done by introducing the
circulationcirculation 
, defined as
with the line integral taken is around a closed circuit C in a counter-clockwise sense. By using Stokes' theorem we may write this as a surface integral,
where the vorticityvorticity 
of the fluid is defined as
If the vorticity of the fluid is zero, we say that the fluid is
irrotationalirrotational:
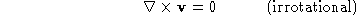
We've already mentioned that there are some useful analogies
between magnetostatics and incompressible fluid flow.
I've collected these together in Table 2.1 below.
To transform the magnetostatics expressions into those for
fluid flow, we make the substitutions 
,
, and 
(where here 
is the mass density of the fluid).
If a fluid is both incompressible and irrotational, then
and 
, which
are the same as the equations of electrostatics and magnetostatics
in free space (i.e., in the absence of charges and currents).
| Magnetostatics | Incompressible fluid flow | |
 | | |
| (Ampére's Law) | (circulation) | |
| (energy density of the magnetic field) | (kinetic energy density of a fluid) |
As an example of fluid flow with vorticity, we can consider a
single vortexvortex, which would be produced
when draining a bathtub, and which is illustrated in Fig. 2.5.
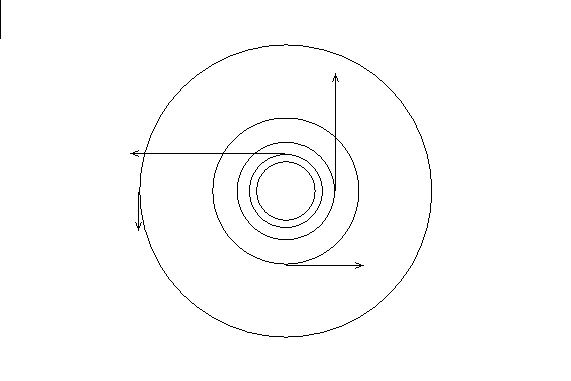
Figure 2.5: Velocity field of a vortex.
Flow lines are drawn for equal increments of the speed, as shown by arrows. Click here to see animations.
In cylindrical coordinates
there is only one component of the velocity field, 
. In
calculating the circulation, the line element
,
so that 
. If the circulation
is independent of the integration path, then we must have 
,
with C a constant. The circulation is then
so that 
. Therefore, the velocity field of a vortex is
By making the substitutions suggested above, we can use this result to find the magnetic field produced by a wire carrying a current I:
